Description
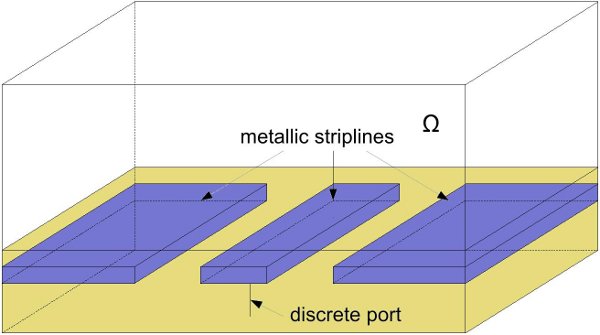
A coplanar waveguide (see Fig. 1) is a microwave semiconductor device, which is governed by Maxwell's equations.
The coplanar waveguide considered with dielectric overlay, i.e. a transmission line shielded within two layers of multilayer board with  thickness are buried in a substrate with
thickness are buried in a substrate with  thickness and relative permittivity
thickness and relative permittivity
 and relative permeability
and relative permeability  , and low conductivity
, and low conductivity  .
The low-loss upper layer has low permittivity
.
The low-loss upper layer has low permittivity  and
and  .
The whole structure is enclosed in a metallic box of dimension
.
The whole structure is enclosed in a metallic box of dimension  by
by  by
by  .
The discrete port with
.
The discrete port with  lumped load imposes
lumped load imposes  current as the input to the one side of the strip.
The voltage along the discrete port 2 at the end of the other side of coupled lines is integrated as the output.
current as the input to the one side of the strip.
The voltage along the discrete port 2 at the end of the other side of coupled lines is integrated as the output.
Data
Considered parameters are the frequency  and the width
and the width  of the middle stripline.
of the middle stripline.
The affine form  can be established using
can be established using  affine terms.
affine terms.
The discretized bilinear form is  , with matrices
, with matrices  .
.
The matrices corresponding to the bilinear forms  as well as the input and output forms and H(curl) inner product matrix have been assembled
using the Finite Element Method, resulting in 7754 degrees of freedom, after removal of boundary conditions. The files are numbered according to their
appearance in the summation.
as well as the input and output forms and H(curl) inner product matrix have been assembled
using the Finite Element Method, resulting in 7754 degrees of freedom, after removal of boundary conditions. The files are numbered according to their
appearance in the summation.
The coefficient functions are given by:
The parameter domain of interest is ![\omega \in [0.6, 3.0] \cdot 10^9 \, \text{Hz}](/morwiki/images/math/f/f/7/ff71040c4c32ee9fc2e11e271a6b8950.png) , where the factor of
, where the factor of  has already been taken into account
while assembling the matrices, while the geometric variation occurs between
has already been taken into account
while assembling the matrices, while the geometric variation occurs between ![\nu \in [2.0, 14.0]](/morwiki/images/math/7/5/6/756a434dfb914a085ee3854e8accd924.png) .
The input functional also has a factor of
.
The input functional also has a factor of  .
.
There are two output functionals, which is due to the fact that the complex system has been rewritten as a real symmetric one.
In particular the computation of the output
 with complex vector
with complex vector  turns into
turns into  with real vector
with real vector  .
.
Origin
The models have been developed within the MoreSim4Nano project.
Data
The files are numbered according to their appearance in the summation and can be found here: Matrices_cp.tar.gz.
Dimensions
System structure:
System dimensions:
 ,
,
 .
.
Citation
To cite this benchmark, use the following references:
- For the benchmark itself and its data:
- The MORwiki Community, Coplanar Waveguide. MORwiki - Model Order Reduction Wiki, 2018. http://modelreduction.org/index.php/Coplanar_Waveguide
@MISC{morwiki_waveguide,
author = {{The MORwiki Community}},
title = {Coplanar Waveguide},
howpublished = {{MORwiki} -- Model Order Reduction Wiki},
url = {https://modelreduction.org/morwiki/Coplanar_Waveguide},
year = {2018}
}
- For the background on the benchmark:
@ARTICLE{morHesB13,
author = {Hess, M.~W. and Benner, P.},
title = {Fast Evaluation of Time-Harmonic {M}axwell's Equations Using the Reduced Basis Method},
journal = {{IEEE} Trans. Microw. Theory Techn.},
volume = 61,
number = 6,
pages = {2265--2274},
year = 2013,
doi = {10.1109/TMTT.2013.2258167}
}
References
- ↑ M.W. Hess, P. Benner, "Fast Evaluation of Time-Harmonic Maxwell's Equations Using the Reduced Basis Method", IEEE Transactions on Microwave Theory and Techniques, 61(6): 2265--2274, 2013.


